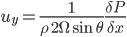The most fundamental reason that Earth has wind is because it is a sphere (basically; it "bulges" slightly at the equator due to its rotation). Differential heating creates the winds, which are then rerouted by Earth's rotation and land-sea boundaries. In turn, winds influence precipitation patterns and indirectly drive ocean currents. This post will walk through the basics. If you don't want to read the whole thing, you can skip to the summary.
Table of Contents:
Solar Input
Luminosity
The total solar radiation that a planet receives depends on the planet's size and distance from the sun. The sun's luminosity (L0)—the energy it releases from its photosphere (visible surface) per unit time—can and does vary, but on average, it is 3.9 × 1026 W (J/s).
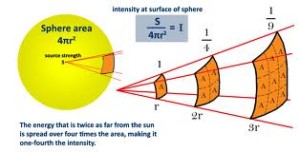
Figure 1. The farther away you move from an energy source, the less concentrated the energy. Image credit: UC Santa Barbara.
To calculate how much of this energy reaches the earth, we need to know the surface area of earth's orbit. Imagine the sun as broadcasting its energy in a sphere around it: the father away you move, the larger that sphere gets. If the same amount of energy (L0) is spread across a larger sphere, then each unit area of the sphere is receiving less energy than would a smaller sphere (Fig. 1). The energy gets "diluted", so to speak.
Solar Constant
The surface area of a sphere is 4πr2, where r is the radius of the sphere. If we plug in the average distance of the sun from the earth (remember, since the earth's orbit is elliptical, this distance varies, but the average is about 1.5 × 1011 meters), and then divide L0 by this value, we can calculate how "diluted" the sun's energy is by the time it reaches the earth. The result is called the solar constant (S0): the amount of energy hitting each unit area of a sphere the size of earth's orbit.
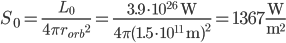
To calculate how much of this energy the earth actually captures, we multiply this value by the area of a circle with earth's radius, which is about 6,370 km (the area of a circle is πr2). We do this because when you are looking straight at the earth, it presents as a circle; solar energy "sees" the earth in the same way, so the energy hits only the circle facing it.
Due to radiative transfer, solar radiation intercepted by the earth will eventually spread across the entire surface area of the earth. So we can divide our value by the surface area of the entire earth (a sphere) to see how much energy is allotted to each unit area. Notice that the πre2 cancel out.
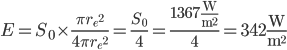
Albedo
Since there are very few buffers in space, virtually all of this actually energy reaches the top of the earth's atmosphere. However, on average, the earth reflects back about 30% of this energy; this reflection is known as albedo, and varies by surface (white and smooth surfaces reflect more—one reason the poles, which are covered in ice, are able to stay cold!). Clouds reflect about 20% of solar radiation, while atmospheric particles reflect about 6%.
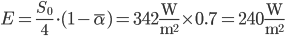
Temperature
We can actually calculate the theoretical temperature (T) of the earth using this energy flux value (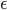 ) and the Stefan-Boltzman constant (
) and the Stefan-Boltzman constant ( ).
).
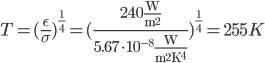
The observed temperature of the earth is 288 K (15 °C), which isn't that far off from our calculation! What makes the real temperature higher than the calculated one? The earth is a greenhouse planet, so it actually stores some energy. (The energy provided from the earth's warm interior is negligible at just 0.06 Wm2.) Over long time scales, though, the energy in is equal to the energy out, or the planet would constantly be increasing or decreasing in temperature.
Bonus: You can also use the energy flux value (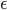 ) multiplied by the surface area to calculate luminosity!
) multiplied by the surface area to calculate luminosity!
Differential Heating
Local Irradiation

Figure 2. Higher latitudes receive less concentrated solar radiation than lower latitudes. Image credit: Hendrix, University of Oregon.
Since the earth is a sphere, the equator receives energy per unit area than do higher latitudes. In other words, at the poles, the same amount of energy is distributed over a greater area. This occurs because the surface area of the plane perpendicular to the sun becomes smaller as you approach the poles (Fig. 2). At the equator, the part of the earth facing the "sun" is flat, but at the poles, the sun has to "reach farther". If the earth was a cube, this wouldn't happen, and all parts of the cube facing the sun would receive the same radiation.
Note that since the earth's axis of rotation is actually titled by 23.5° with respect to the sun, the sun only directly hits the geographic equator during the equinoxes (Fig. 3). The the latitude that the sun actually directly hits at noon is called the solar equator, and represents the latitude of maximum solar radiation. During the solstices, the sun hits the solar equator at either 23.5° N or 23.5° S. The declination of the sun (δ) is the angular difference between the geographic equator and the solar equator, and is 0° during the equinoxes and ±23.5° during the solstices. The solar zenith angle (ϕ) is the angle between the sun and the local vertical and is equal to the latitude minus the declination (ϕ = θ - |δ|). During the equinoxes, this angle is equal to the latitude. The solar zenith angle is always 0° at the solar equator. The solar zenith angle is also called the effective latitude as it controls how much solar radiation an area receives.
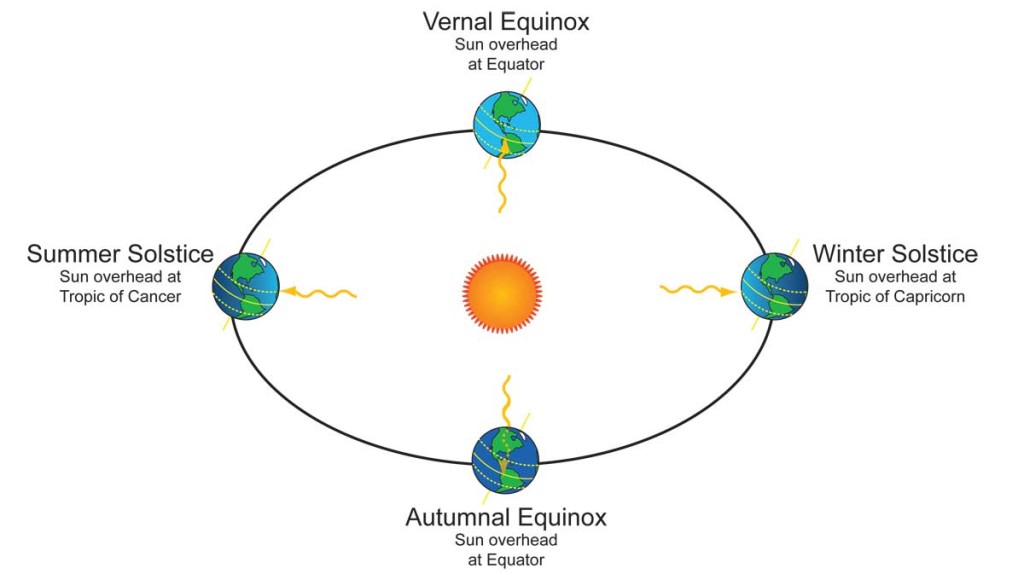
Figure 3. The earth's axis of rotation is tilted with respect to the sun, so depending on the season, the latitude directly hit by the sun changes. Image credit: NASA.
With this knowledge, we can calculate local irradiance: the amount of solar radiation hitting a specific latitude. The method is similar to how we calculated the solar radiation that each location of the earth receives on average. Let's re-think this equation in terms of the cross-sectional area being hit by the sun (the circle) to the total area (the sphere):
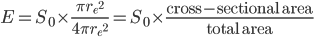

Figure 4. The solar zenith angle, the angle between the sun and the local vertical, affects how much solar radiation an area receives. mImage credit: C. Cameron.
So how can we calculate the cross-sectional area and total area of any specific point on earth? Trigonometry allows us to use the solar zenith angle (the angle between the sun and the local vertical). If you imagine an infinitely small point on the surface of the earth at a given latitude, the ground represents the surface area, and the line perpendicular to the sun's ray represents the cross-sectional area (Fig. 4). These two lines intersect to form a right triangle such that the cross-sectional line is the hypotenuse and the surface line is the adjacent limb. Since the cosine of an angle is equal to the adjacent divided by the hypotenuse, we can use the cosine of the solar zenith angle (cos ϕ) to calculate the ratio between the cross-sectional area and the total area. We need to also need to account for the local albedo, which can have a great impact on how much energy stays at the surface (albedo is significantly higher at the poles, further reducing temperatures there).
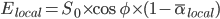
Seasonal Variability
The titled axis of the earth explains the seasons. When the Northern Hemisphere is tilted toward the sun, the Northern Hemisphere experiences summer while the Southern Hemisphere experiences winter (Fig. 3). When the Northern Hemisphere is tilted away from the sun, the Northern Hemisphere experiences winter while the Southern Hemisphere experiences summer. The tilt of the earth helps to distribute heat to higher latitudes: without the tilt, the temperature gradient between the poles and equator would be even more dramatic!
If every place on earth experiences the same ±23.5° swing each year, why do the tropics maintain relatively constant in temperature year-round while higher latitudes can swing from hot summers to freezing winters? Because local irradiance depends on the cosine of the solar zenith angle. As you can see in Figure 5, the change in the value gets much steeper as you approach 90°. A 5° change from 10° to 15° results in a 1.9% decrease in radiation. However, a 5° change from 75° to 80° results in an 8.5% decrease!
Winds & Precipitation
Atmospheric Cells
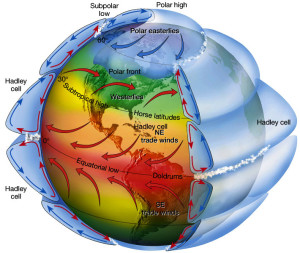
Figure 6. Each hemisphere has three atmospheric cells, driven by differential heating and rotation of the earth. Image credit: Lutgens and Tarbuck, The Atmosphere.
Differential heating—with a hot equator and cold poles—creates a pressure gradient between the equator and poles. Since warm air is less dense, warm air at the equator rises and travels poleward, where it sinks. To fill the space left behind, air from the higher latitudes the rushes toward the equator.
On a non-rotating planet, there would be just one gigantic atmospheric cell in each hemisphere. However, since earth rotates, such a large cell isn't stable, and it breaks down into three cells. The Hadley cells, which are the strongest, cover the equator from roughly 0° to 30°. The Ferrel cells lie between 30° and 60°. The polar cells, which are the smallest, stretch from 60° to 90°. Air approaches and rises at latitudes 0° and 60° (low pressure areas), while air sinks and then travels away at latitudes 30° and 90° (high pressure areas).
Remember, though, that since the solar equator experiences a 46° swing, these cells—which are driven solely by heat differences between the equator and the poles—will also migrate (Fig. 7). This is why the jet streams, which form between the cells, have different locations in summer and winter. The jet streams intensify in the winter when the temperature gradient between the equator and poles is greater. The Intertropical Convergence Zone (ITCZ), also called the doldrums, falls between the Hadley cells and also moves with the seasons; since there is more land mass in the Northern Hemisphere compared to the Southern Hemisphere, and land tends to be hotter than water, the ITCZ tends to be positioned slightly northward.
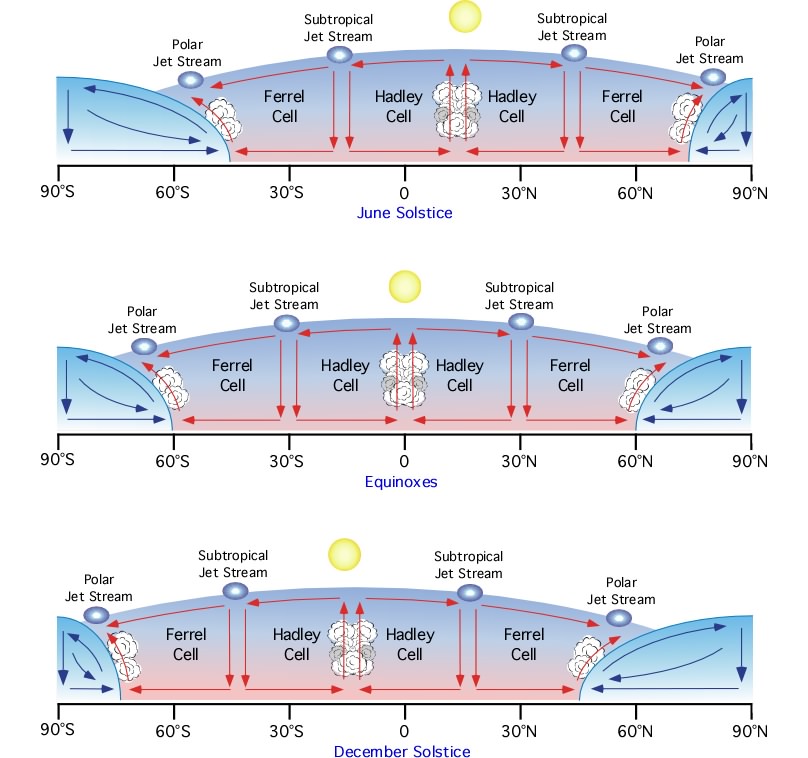
Figure 7. The position of the cells changes with the solar equator. Image credit: PhysicalGeography.net
Global Precipitation Patterns
Warm air holds more water vapor than does cold air, and higher altitudes are colder than lower altitudes. As air is rising near the equator and at 60° latitude, it cools in the atmosphere; cold air holds less water, so the water vapor condenses and falls as precipitation (Fig. 8). This why the tropics are rainy. On the other hand, at around latitude 30°, the water-depleted air sinks and reaches warmer temperatures; now that the air can hold more water, evaporation occurs. Due to the evaporation, the atmosphere is rich in moisture at 30°, so water follows the moisture gradient and moves toward 60° and 90°—which makes precipitation even less likely around 30°. Since evaporation exceeds precipitation around 30°, deserts tend to form along this latitude, as in Africa and Australia (Fig. 9). You might notice the distinct lack of desert in the heavily forested southeastern United States, even though it surrounds prime desert latitude; this is because a local high pressure system forces moist warm winds over the region.

Figure 8. Atmospheric cells and low and high pressure areas control which areas experience more precipitation than evaporation. Image credit: Encyclopedia Britannica, EOEarth.
The Coriolis Effect
The Earth's rotation does more to our winds than break them down into three cells. As air moves in north-south directions, the planet rotates from west to east beneath it. Imagine an air parcel traveling southward from around Iceland, heading for Africa; by the time it reaches the equator, the earth has rotated beneath it, and it lands in the Pacific Ocean—west of its target—instead (Fig. 10).
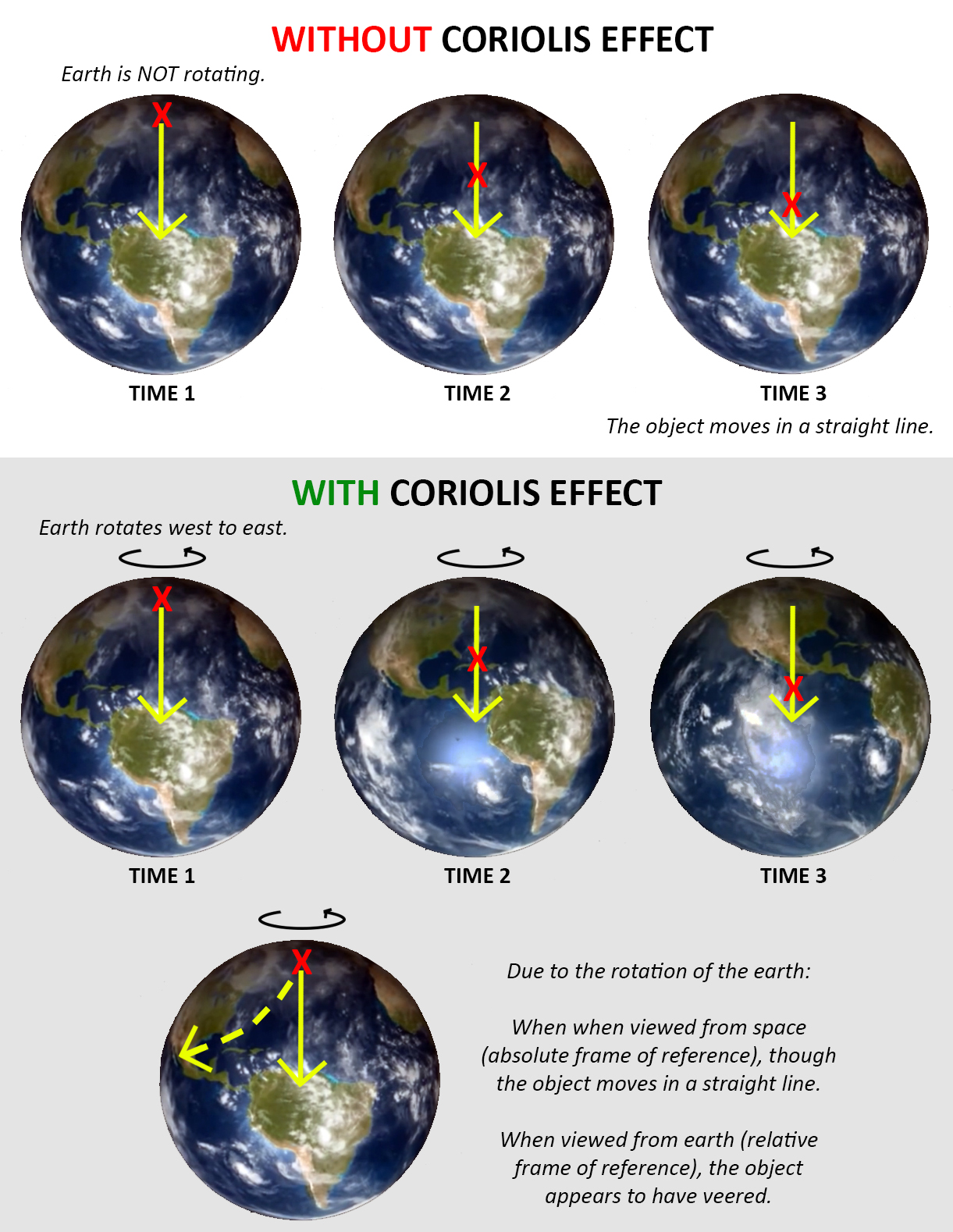
Figure 10. Due to the Coriolis effect, an object moving from Iceland to Africa is "deflected" into the Pacific Ocean. Image credit: C. Cameron.
This phenomenon is called the Coriolis effect, which deals with the apparent deflection of objects moving on a rotating body. The deflection occurs because the earth at the equator is wider than at the poles, so the equator has to spin faster to keep up. The Coriolis effect is greatest at the poles and slowly loses strength as you move to lower latitudes until it equal to zero at the equator. In fact, movements directly over the equator are not deflected at all. Even objects moving in an east-west direction are deflected. This is because the surface of the earth is curved, not flat, so there is always a component of large-scale movements that is north-south.
However, the deflection only occurs from a relative frame of reference. As shown in Figure 9, if you are in space when you view the air parcel moving, then it appears to travel in a straight line. However, if you are on the rotating the body (the earth), and you see a southward-moving parcel that starts out near Iceland end up in the Pacific, then it stands to reason that the parcel veered westward.
The most important things to remember about the Coriolis effect:
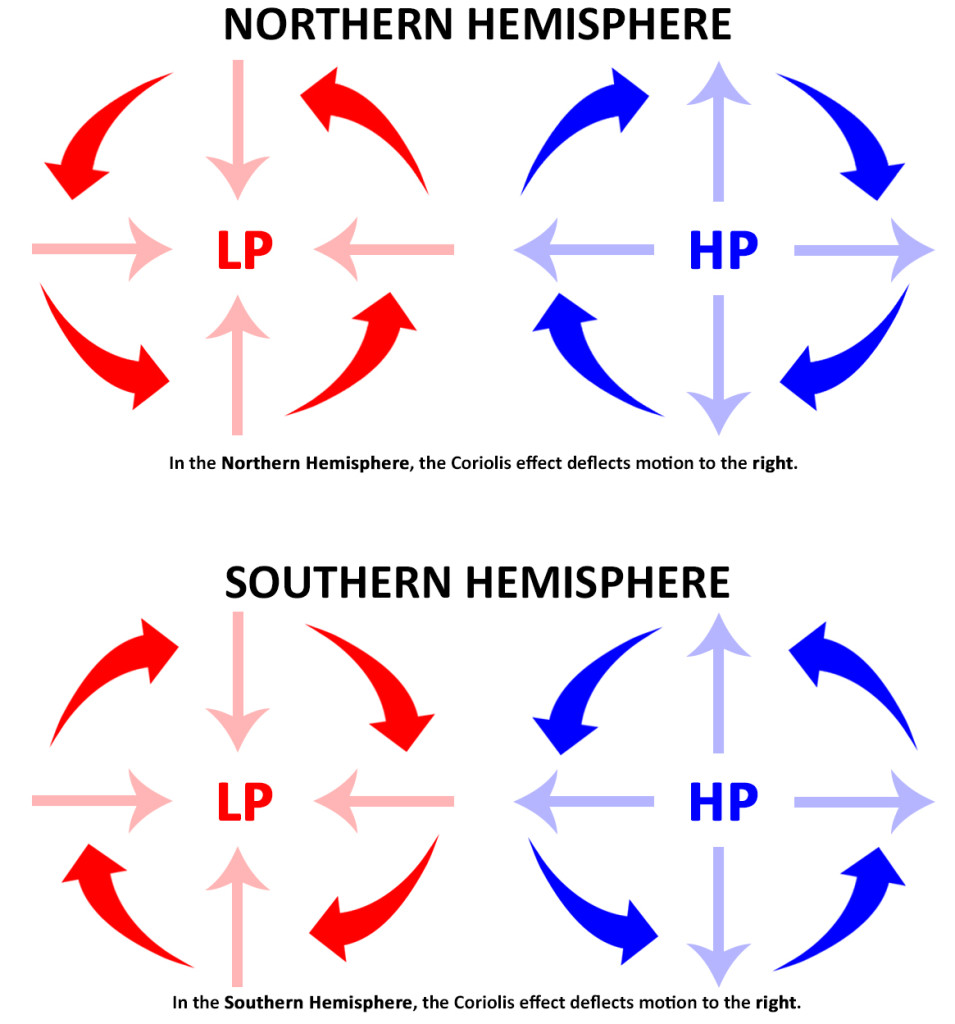
- Due to the west-to-east rotation of the earth, in the Northern Hemisphere, the Coriolis effect will deflect motions to the right. In the Southern Hemisphere, it will deflect motions to the left.
- The Coriolis effect acts perpendicular to an object's movement and acts perpendicular to the axis of the earth's rotation.
| The Coriolis Effect | ||
| Motion | Deflection to the Right in Northern Hemisphere | Deflection to the Left in Southern Hemisphere |
| Eastward | Southward | Northward |
| Westward | Northward | Southward |
| Northward | Eastward | Westward |
| Southward | Westward | Eastward |
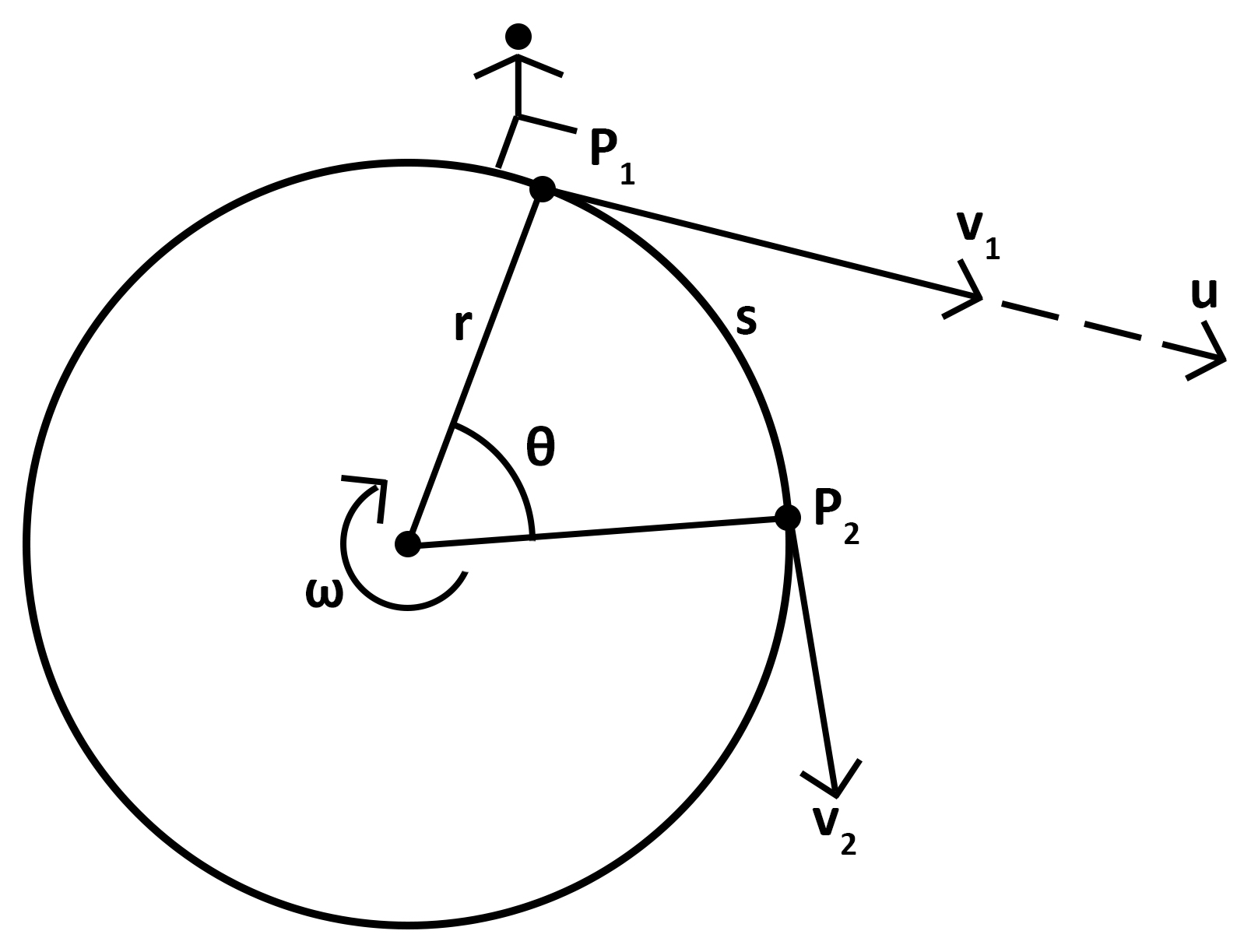
Now let's work out how to mathematically calculate the strength of the Coriolis effect at any given latitude. In Figure 11, r is the radius, ω is the angular velocity (how long it takes to complete one revolution), v is the tangential velocity created by the rotation (calculated by ω × r), and u is the person's walking speed.
Intuitively, we know that the speed that the person is traveling depends on how fast the merry-go-round is spinning. We also know that on a smaller merry-go-round, the speed will be less than on a large merry-go-round. Therefore, using dimensional analysis, we can discover the tangential velocity (v1):
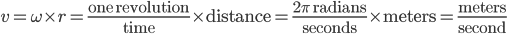
Since the person is walking, we need to add their velocity (u, also in meters per second) to the total:
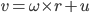
The acceleration of a centripetal force is equal to:
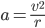
If we substitute for v and expand:
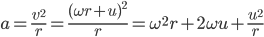
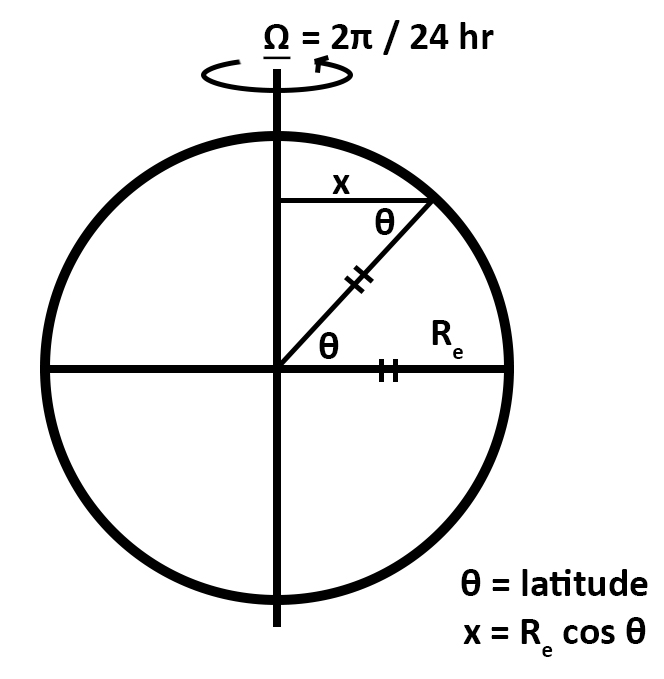
How can use this equation for each point on the earth? We know that the radius of the earth is about 6,370 km, but using that value would only give us the Coriolis effect at the geographic equator. Why? If you imagine each latitude as a spinning disk, you see that higher latitudes have smaller "disks" than lower latitudes, so they have smaller radii (in Fig. 12, Re > x). Fortunately, trigonometry allows us to calculate the radius of each latitude's disk. We can exploit the fact the distance from the center of a sphere to any point (the radius) on the circle is equal. Figure 12 shows how the latitude relates to the size of that latitudes disk: we simply multiply the radius of the earth by the cosine of the latitude (x = Re cos θ).
For earth, the equation is typically written like this (it is equal to zero because the net acceleration of objects experiencing the Coriolis effect is zero; otherwise, the winds would constantly be gaining speed):
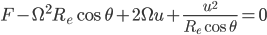
Keeping in mind that Ω is one revolution every 24 hours and that Re is 6,370 kilometers, let's consider each term in this equation:
- F stands for the real forces acting on the body, such as pressure gradient forces, which we'll discuss later; since the other terms aren't real forces so much as they are the result of a rotating frame of reference, they're called pseudo-forces.
- Ω2 Re cos θ is the centripetal acceleration that results from being on the earth and is directed radially outward; it acts against gravity, but it's extremely small compared to gravity (about 0.1% the magnitude), so it folds into gravity.
- u2/Re is another centripetal acceleration that arises from an object's own movement, but this force is negligible; based on scaling analysis, which compares the importance of this term to the other terms, it doesn't begin to have an impact until an object reaches 3,500 km/hr (the fastest observed wind speed on earth was 370 km/hr).
- 2Ωu is the Coriolis effect that results due to an object's own movement.
Since the Coriolis effect acts
- perpendicular to the rotational axis of the earth, and
- perpendicular to the motion of the object,
it can be understood as the cross-product between the earth's rotation and an object's velocity.
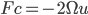
We can break down the rotation of the earth (Ω) into three components: one each along the east-west (x), north-south (y), and up-down (z) directions.
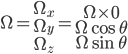
We must also consider the object's own velocity and how it is split up between the x, y, and z directions.
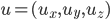
Now we simply need to find the cross-product. Note that i, j, and k are unit vectors for the x (east-west), y (north-south), and z (up-down) directions, respectively.
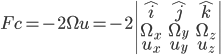
This confirms that, as we saw in Figure 10, motions in the north-south direction cause an east-west deflection. Motions in the east-west direction cause a north-south deflection.
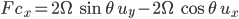
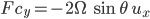
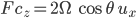
However, for wind and ocean currents, the vertical (up-down) direction is basically negligible:
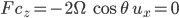
If we substitute this value into our equation for the Coriolis effect on east-west motions, we end up with the two equations we'll be needing when we look at geostrophic flow (a type of ocean current):
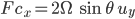
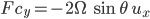
Global Wind Patterns
The result of the Coriolis effect on winds is that north-south trending winds are deflected to the east and west (Fig. 13)! On a non-rotating planet, the wind would basically travel north and south, as shown in our model of atmospheric cells (Fig. 7); however, since our planet rotates, the winds experience the Coriolis effect.
Remember these three things, and it is very easy to map out the global wind pattern:
- where the high and low pressure areas are (recall the warm air at the equator rises, creating a low pressure point, and work your way forward from the that)
- that air rushes away from the high pressure areas and toward to the low pressure areas
- movements are deflected to the right in the Northern Hemisphere and to the left in the Southern Hemisphere.
Winds are named for the direction that they are coming from, NOT for where they are going. For example, a wind traveling to the east is called a westerly. The northern and Southern Hemisphere wind belts mirror each other. I find that it is easy to remember the order of the winds by thinking EWE for Easterlies, Westerlies, then Easterlies. Easterlies travel toward the equator (and that's easy to remember because they both start with "E").

Figure 13. The Coriolis effect gives a zonal (east-west) component to the movement of winds. Image credit: Lutgens and Tarbuck, The Atmosphere.
Northern Hemisphere
- 90° N - 60° N: Polar easterlies
(southeast trending) - 60° N - 30° N: Westerlies
(northwest trending) - 30° N - 0°: Trade winds
(southeast trending)
Southern Hemisphere
- 0° - 30° S: Trade winds
(northwest trending) - 30° S - 60° S: Westerlies
(southeast trending) - 60° S - 90° S: Polar easterlies
(northwest trending)
Land-Sea Differences
Water has a high heat capacity, which is the amount of energy needed to raise a unit of mass by 1°C. Here are the heat capacities of air, soil, and water:
- Air: 1.2 × 103 J·m-3·°C-1
- Soil: 2.8 × 106 J·m-3·°C-1
- Water: 4.2 × 106 J·m-3·°C-1
As you can see, compared to other materials, water is resistant to temperature changes. Therefore, land heats up and cool downs much more rapidly than does water. During the day, land is warmer than water, because land gains heat faster, but at night, land is cooler than water, because land also loses heat faster.
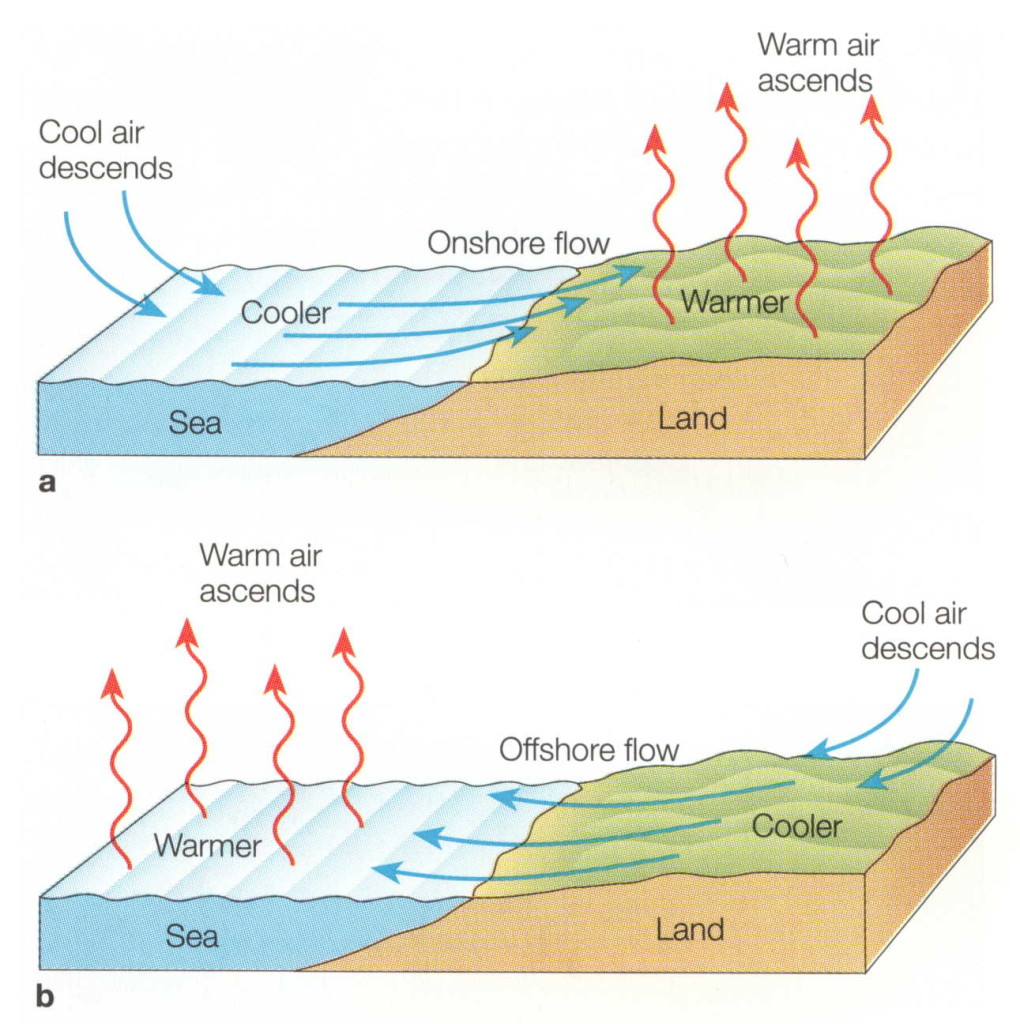
Figure 14. During the day, when land is hotter than sea, the warm air over land rises, causing wind to move onshore. Image credit: Garrison, 1993.
This creates a pressure gradient between the land and the sea, much like we saw happening between the equator and the poles. During the day, when the land is warmer, the air rises, creating a low pressure spot; air rushes in toward the land from over the sea, creating onshore winds (Fig. 14). At night, the opposite occurs; the land temperature drops, and the air over the land rushes out toward the sea, creating offshore winds.
Why does water resist temperature change? First, it can move fluidly, allowing it to redistribute heat. Land is limited to conduction, which is incredibly slow. Second, water is a polar molecule with a more negative side and more positive side, which causes water molecules to attract each other like magnets (the positive end of one water molecule will be attracted to the negative end of another). This special property of water is called hydrogen bonding. Due to the intermolecular attraction between part molecules, more energy is needed to stir up the molecules and heat them!
Atmospheric Gyres
Land-sea differences combine with the Coriolis effect to create atmospheric gyres.
At cool high latitudes, the sea tends to stay warmer than the land, creating a low pressure area over the sea that air rushes toward. When the land gets even colder in winter, the gyre intensifies. At warm low latitudes, the land tends to sea warmer than the sea, which creates a high pressure area over the sea that air rushes away from. When the land gets even warmer in summer, the gyre intensifies.
This general east-west movement of the air between the land and sea is deflected by the Coriolis effect. Air begins circling around the low/high pressure area over the sea, a gyre forms (Fig. 15). In the Northern Hemisphere, low-pressure gyres rotate counterclockwise (cyclonic), while high-pressure gyres rotate clockwise (anticyclonic). In the Southern Hemisphere, low-pressure gyres rotate clockwise (cyclonic), while high-pressure gyres rotate counterclockwise (anticyclonic).
Land-sea differences and the Coriolis effect complicate the patterns of our wind (Fig. 16). On a global scale over very long time periods, the winds resemble the idealistic patterns that we mapped out based on differential heating, low and high pressure areas, and the Coriolis effect. However, over short time scales and at small spatial resolutions, the winds can be quite idiosyncratic, changing in strength and direction on a day-to-day or even hourly basis.
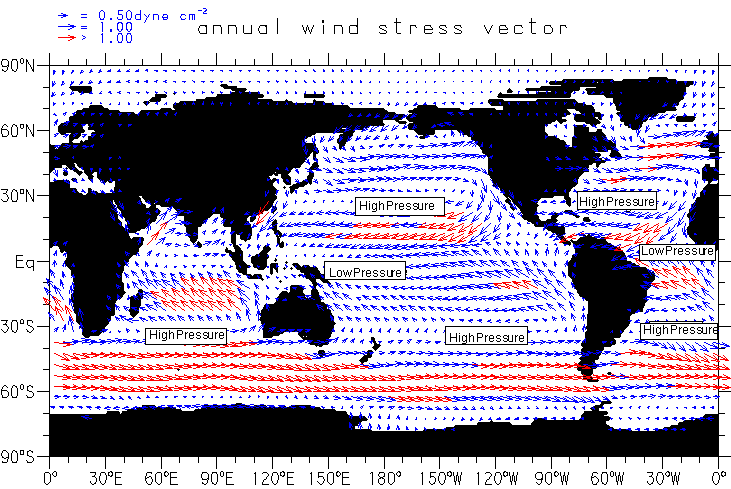
Figure 16. Global map of the wind direction and strength based on annual averages. Image credit: Don Reed, San José State University.
Instabilities & Transients
Due to differential heating, the atmosphere slopes such that poles are under greater atmospheric pressure than the equator (Fig. 17). The sun is constantly trying to exacerbate the gradient. The pressure gradient force is always trying to erase the gradient. Any perturbation causing motion perpendicular to an isopycnal—a line of equal pressure—will produce an acceleration. These so-called instabilities are an attempt to decrease meridional (north-south) temperature and density gradients. The constant battle between these two forces helps to explain why day-to-day weather in certain areas (such as North Carolina) can vary so much, because the atmosphere experiences meanders where colder air is able to force its way in. To sum up, instabilities are driven by density gradients. In the next section, we'll talk about pressure gradient forces, which determines the fate of movements by instabilities.
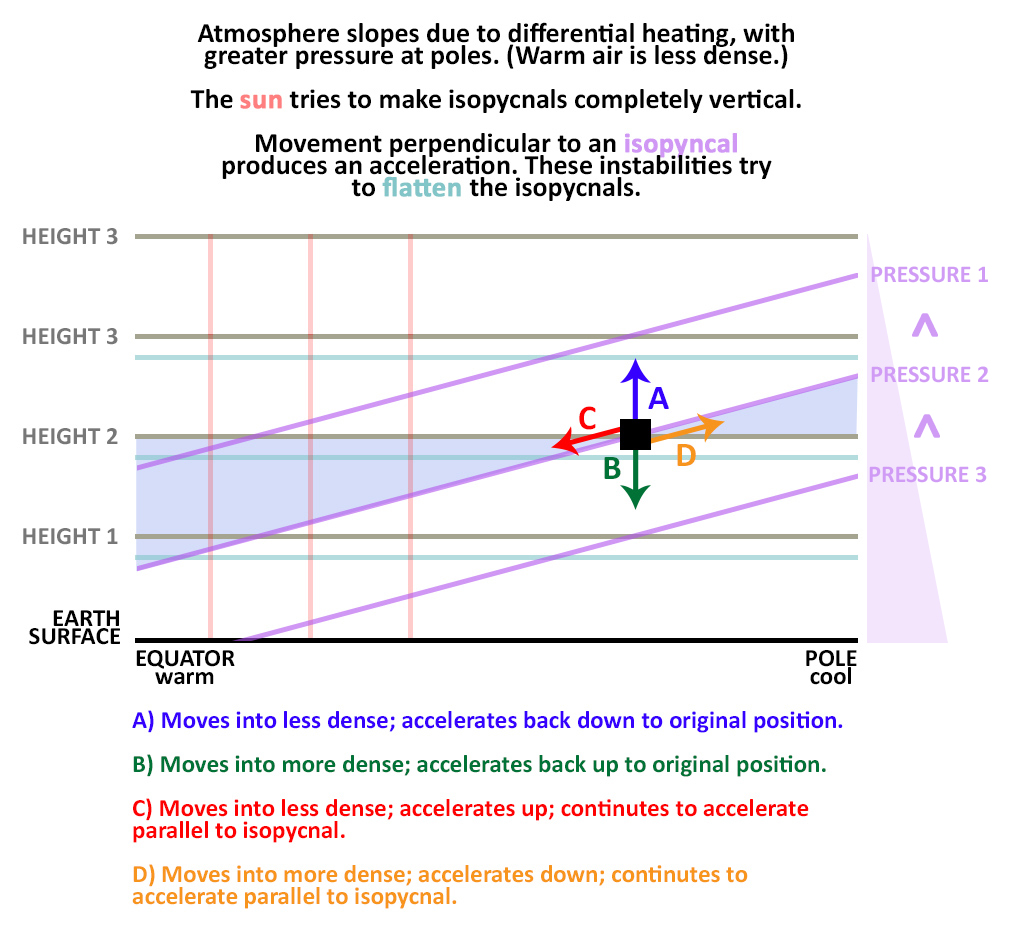
Figure 17. The poles are under greater atmospheric pressure than the equator due to differential heating: the atmosphere slopes. Image credit: C. Cameron.
Ocean Currents
Pressure Gradient Forces
In nature, things tend to move from more to less in an attempt to even things out; this is why wind forms in response to temperature gradients that result from differential heating of the earth. In the ocean, water moves from areas of more pressure to areas of less pressure.
The pressure (P, in kg·m-1·s-2) exerted on a point in the ocean is calculated by multiplying the height (h, in m) of the water column over the point, the density (ρ, kg·m-3) of the water in the column, and the gravitational force (g = 9.8 m·s-2) acting on the water column.
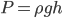
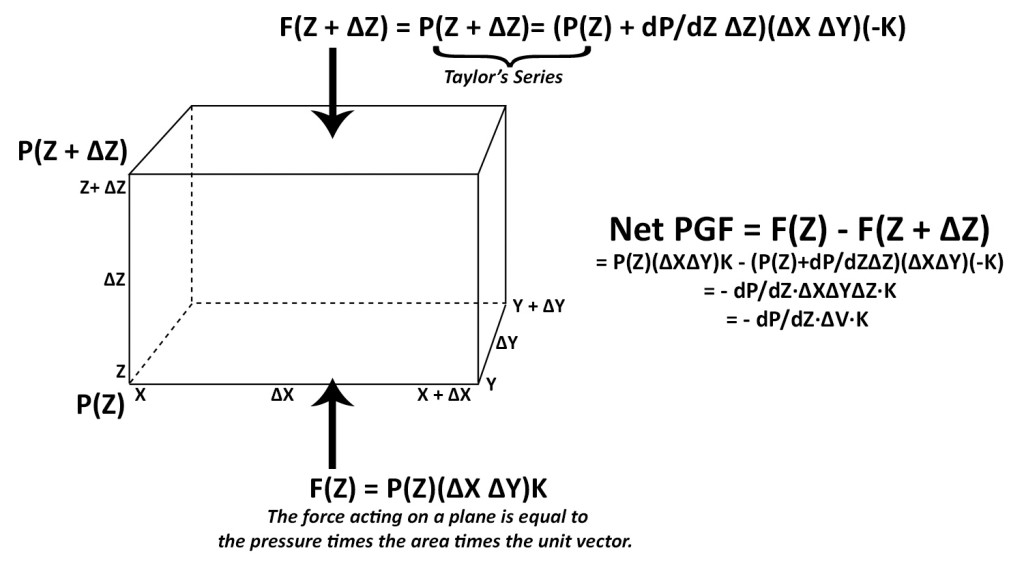
We can find the net pressure gradient force acting on a fluid parcel (a box of fluid) by calculating the difference between two pressures being exerted on opposite sides of the parcel (Fig. 18). The parcel would move in the direction of the lesser force.
For our example, we'll imagine that pressures are being exerted in the vertical direction. The force acting on the bottom of the parcel, F(z), is equal to the pressure (P) at depth z times the area of the plane being acted on (ΔxΔy) times the unit vector (k).
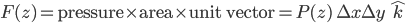
To find the force at depth z + Δz, we'll need to use the Taylor's series, which solves for the value by summing derivatives:
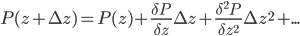
Higher order derivatives become increasingly small, so we can ignore everything after the first derivative. Now we just need to plug in :

To find the difference between the two forces, we simply subtract:
![F(z) - F(z + \Delta) = (P(z)\; \Delta x\Delta y\; \hat{k}) - ([P(z) + \frac{\delta P}{\delta z}\Delta z]\; \Delta x\Delta y\; \hat{k})](http://www.luckysci.com/wp-content/plugins/latex/cache/tex_aec0bc12474592fb999c3bc26c71b878.gif)
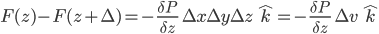
As mentioned, the parcel will move in the direction of the lesser force. Water moves from areas of greater pressure to areas of lower pressure.
We need to consider the mass of the object being acted upon, so we'll divide the equation by the mass. Mass is equal to density times volume, allowing us to rewrite the equation in terms of density.

The above equation applies to forces acting in the vertical direction, but it can be re-worked to apply to any direction using the same procedures.
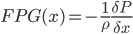
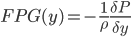
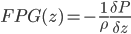
We'll look at the top two equations again when we consider how the Coriolis effect and pressure gradient forces work together to produce geostrophic flows. We'll examine the last equation when we look at the hydrostatic balance.
Density & Hydrostatic Balance
Cold, salty water is denser than warm, fresh water. The water column is stable when less dense water overlies denser water, which is how things usually are in the ocean (Fig. 19). However, when heavier water is on top of lighter water, vertical mixing occurs: surface water sinks to be replaced by rising deep water. Deeper water harbors more nutrients, which overturning brings to the surface where phytoplankton can access them. Vertical mixing happens when air temperatures fall sharply in winter, cooling the surface water. The cold surface water becomes too dense for the immediately underlying waters to support, and it sinks until it reaches the layer of water whose density it matches. Since mixing occurs every winter, it is also called seasonal overturning. The depth to which overturning reaches is called the mixed layer. In the tropics, the mixed layer is shallow (5-20 m) because heavy rain and sunlight keep surface water fresh and warm. In the mid-latitudes, it extends 200-400 meters down. In high latitudes, it reaches 1,000 meters deep.
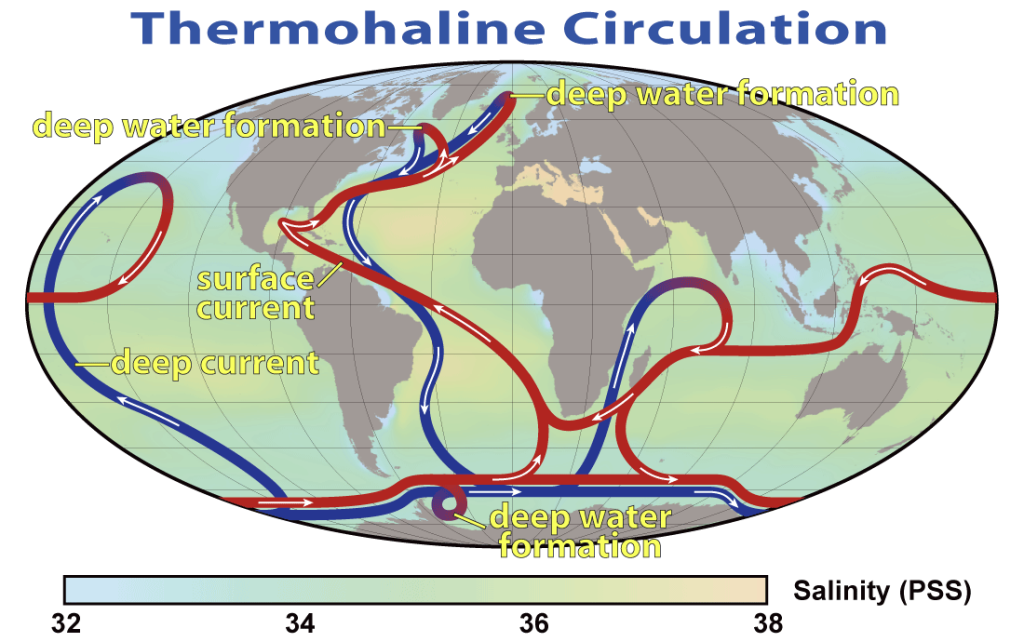
Density differences drive meridional overturning, or global ocean circulation, a 1,000-year cycle whereby water is born deep in the chilly northern Atlantic Ocean, travels to the other ocean basins, surfaces in the Southern and Pacific Oceans, and then heads back to the Atlantic (Fig. 20).
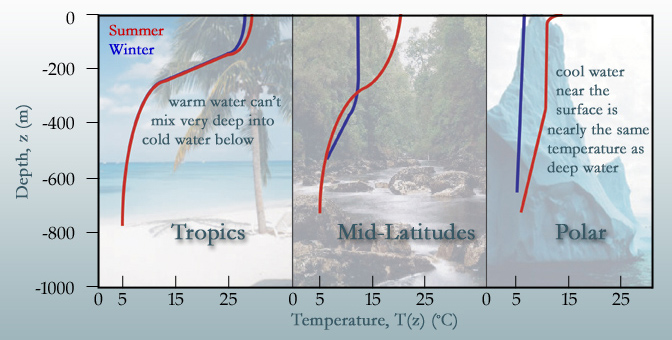
Figure 19. Vertical mixing occurs when surface water becomes more dense than the water below it. Image credit: Iowa State.
We discussed that there is no meaningful Coriolis effect in the vertical direction for atmospheric and ocean movements. Therefore, the pressure-force gradient—based on density—and its interaction with gravity is the driving force behind vertical movements in the ocean. Recall that:
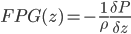
We simply need to set the equation equal to gravity, and we can make calculations on how fluid parcels in the ocean will response to pressure gradients. Gravity is a negative acceleration (9.8 m·s-2). When the two terms are equal, there is no movement. This is called the hydrostatic balance.
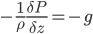
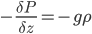
Ekman Transport
Surface currents travel at 45° to the wind due to a balancing act between the current itself, friction—which acts in the opposite direction of the current—and the Coriolis effect (Fig. 21). Remember that in the Northern Hemisphere, the current will travel to the right of the wind, while in the Southern Hemisphere, it will travel to the left of the wind.
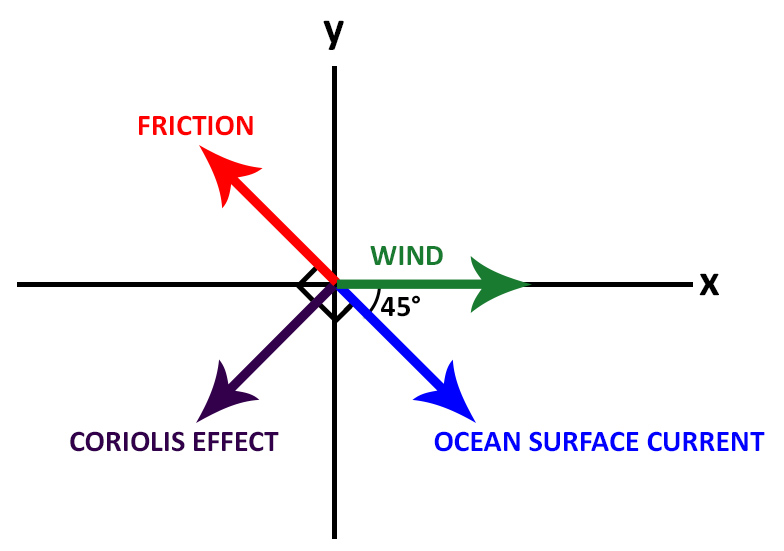
Figure 21. Surface currents travel at 45° to the wind due friction and the Coriolis effect. Image credit: C. Cameron.
At increasingly deeper layers, frictional coupling causes deeper currents to adjust . However, energy dissipates at each successive layer, resulting in slower currents farther down. By integrating all the velocities, we can find that the net movement of ALL water occurs at 90° to the wind (Fig. 22).
This is called Ekman transport, the net transport of water at 90° to the wind due to frictional coupling between successive water layers and the Coriolis effect. In the Northern Hemisphere, the transport is 90° to the right of the wind; in the Southern Hemisphere, the transport is 90° to the left of the wind.
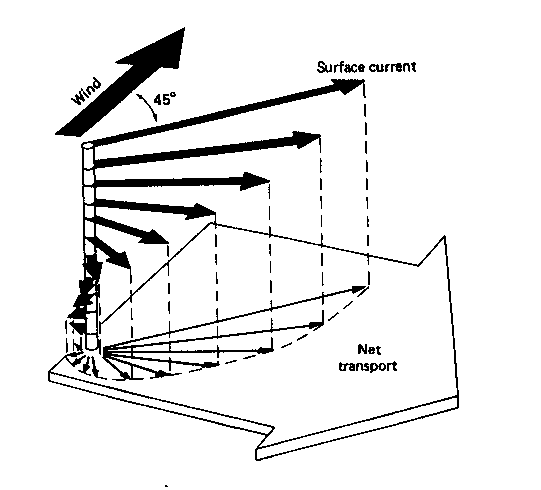
Figure 22. Ekman transport is the net transport of water at 90° to the wind due to frictional coupling and the Coriolis effect. Image credit: San Francisco State University.
Convergence & Upwelling
At certain places on the earth, the winds are positioned such that Ekman transports either combine or separate (Fig. 23). When they combine, convergence causes a pile of water to accumulate. When they separate, divergence causes a depression.
As we discussed previously, a deeper water of column exerts more pressure than a shallower one. Therefore, areas of convergence in the ocean have more pressure than surrounding waters. This creates a pressure gradient force, and water in areas of convergence sink and then move toward the low pressure areas. The sinking is downwelling. As discussed, surface waters are nutrient-poor, because many organisms live there and consume the nutrients, so areas of downwelling support limited life. However, in areas of divergence, water rises; this is known as upwelling. Upwelling provides the surface with nutrient-rich deep waters, which allows phytoplankton to prosper.
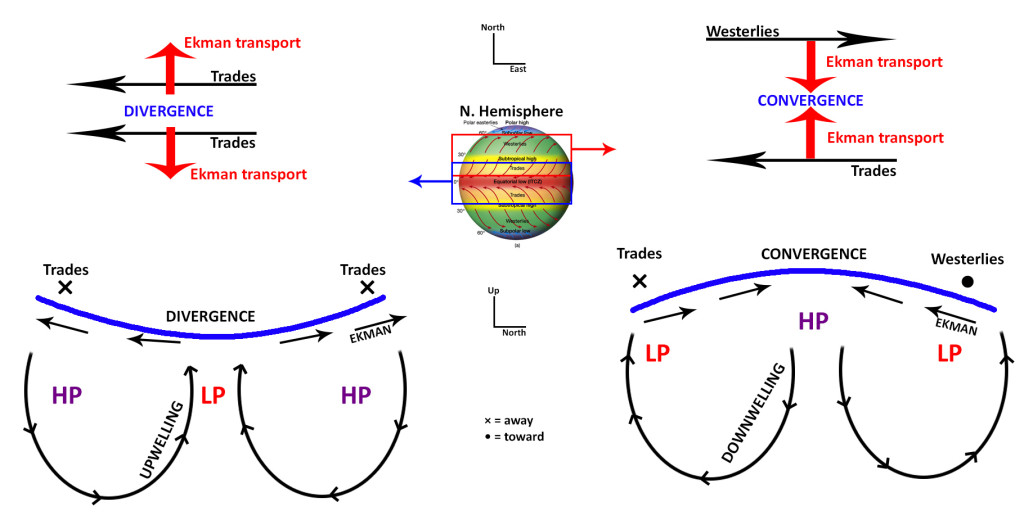
Figure 23. Image credit: Ekman transports produce convergences and divergences, which leads to upwelling and downwelling, respectively. C. Cameron.
Therefore, regions of upwelling—such as the equator (Fig. 23, left)—tend to be very productive and full of animal life. When convergence and divergence cause water to move, that water is then deflected by the Coriolis effect, setting up the pattern of global surface currents. The equator and the poles experience upwelling; the mid-latitudes experience downwelling (Fig. 23, right). However, upwelling can also occur when winds running along the coast cause a seaward Ekman transport, and deeper waters rush up to the coast (Fig. 23). This allows certain coastal regions—like California—that lie along downwelling latitudes to actually instead receive upwelling.
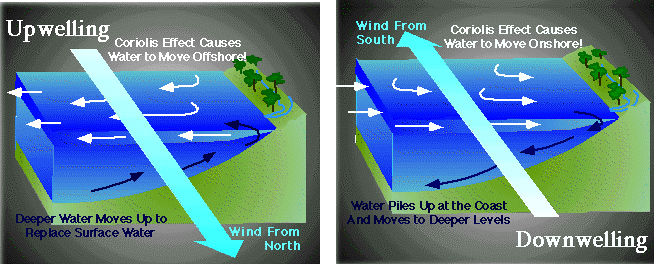
Figure 24. When winds run along coasts, Ekman transports create upwelling or downwelling. The example in this image applies to the Northern Hemisphere. Image credit: crd.bc.ca
| Coastal Upwelling or Downwelling | ||
| Northern Hemisphere | ||
| West Coast | East Coast | |
| Polar Easterlies (90-60°, blow SW) | Upwelling | Downwelling |
| Westerlies (60-30°, blow NE) | Downwelling | Upwelling |
| Trade Winds (30-0°, blow SW) | Upwelling | Downwelling |
| Southern Hemisphere | ||
| West Coast | East Coast | |
| Trade Winds (0-30°, blow NW) | Upwelling | Downwelling |
| Westerlies (30-60°, blow SE) | Downwelling | Upwelling |
| Polar Easterlies (60-90°, blow NW) | Upwelling | Downwelling |
Currents: Geostrophic Flow
Surface currents of the ocean mirror the global wind pattern, but not because the wind itself is moving all the water—although the winds do provide the initial push. Everything we've discussed up until now plays a role in causing currents to follow the direction of winds.
- Winds create Ekman transport.
- Ekman transports causes convergence (or divergence).
- Convergence (or divergence) causes high pressure and low pressure zones in deep waters.
- High pressure and low pressure zones create horizontal pressure gradient forces.
- pressure gradient forces cause water to move horizontally from high to low pressure.
- This horizontal water movement adjusts until it is 90° to the Coriolis effect and traveling at 90° to the pressure gradient force. The Coriolis effect and pressure gradient force are equal but opposite (because the net acceleration is zero; otherwise, winds and ocean currents would constantly be gaining speed).
- The net result is that ocean currents are the same as the direction of the winds (compare Fig. 25 to Fig. 17).
We've covered points 1-5, so let's discuss point 6. The horizontal movement of surface water arising from a balance between the pressure gradient force and the Coriolis effect is known as geostrophic flow. Essentially, the pressure gradient forces and the Coriolis effect cancel each other out.
Since the Coriolis effect acts on any moving object, it acts on water moving due to a pressure-force gradient; the water keeps adjusting (Figs. 26, 27, 28) its direction until:
- The movement of water moving 90° to the pressure-force gradient.
- The pressure-force gradient and Coriolis effect are opposite each other.
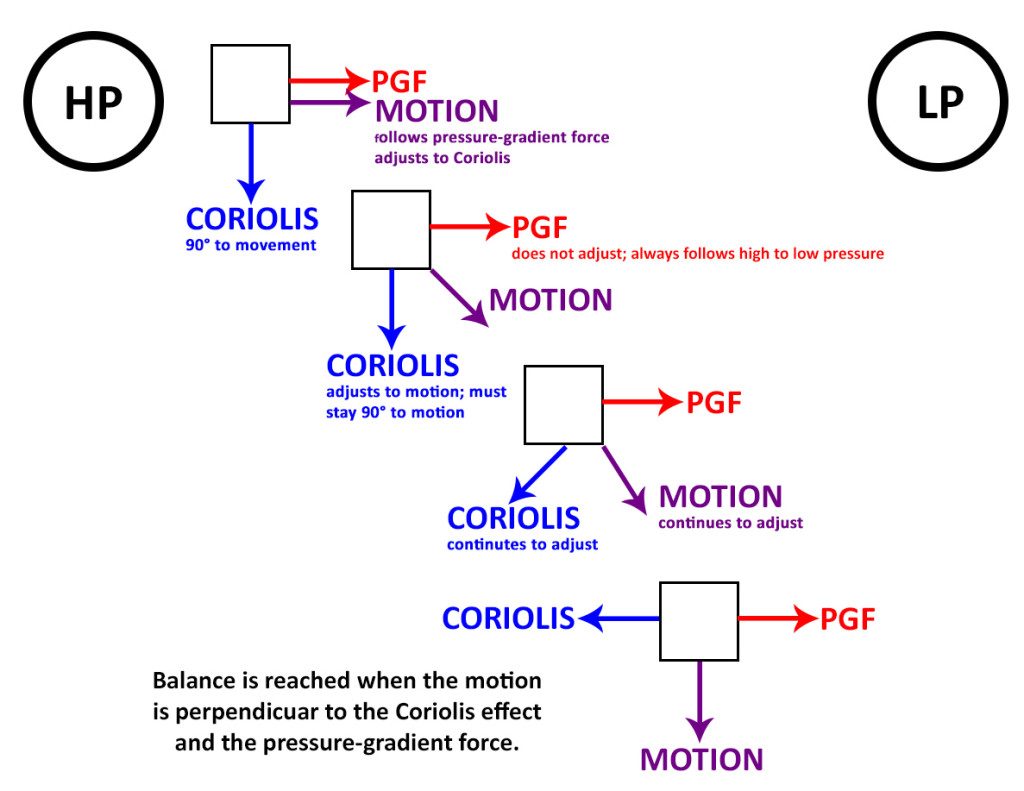
Figure 26. The movement continues to adjust until it is perpendicular to the Coriolis effect and the pressure gradient force. Image credit: C. Cameron.
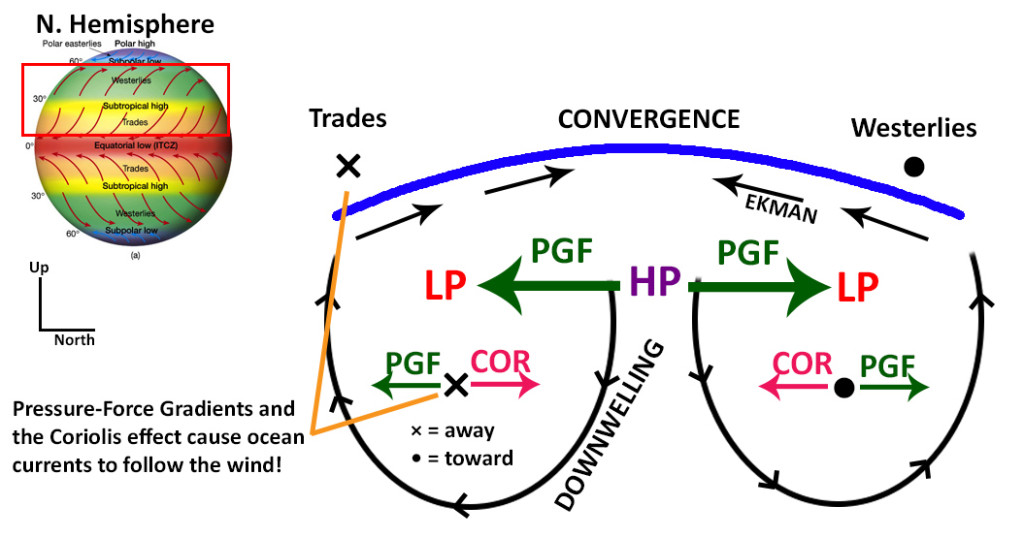
Figure 27. Pressure-force gradients and the Coriolis effect cause ocean currents to follow the wind! Image credit: C. Cameron.
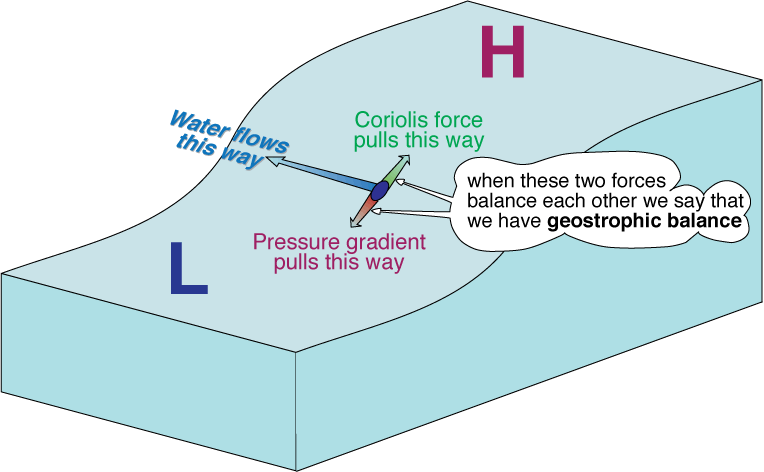
Figure 28. The geostrophic balance causes water to flow 90° to the pressure gradient force. Image credit: seos-project.eu
We found the equations for the pressure-force gradients and the Coriolis effect, and because these two forces cancel each other out (the net acceleration is zero, or winds and ocean currents would constantly be gaining speed), we set them equal to each other. We have found the geostrophic balance for east-west (x) and north-south (y) geostrophic flows.
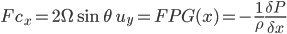
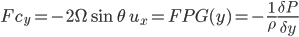
By knowing the density, height, and latitude differences at the ends of a geostrophic flow, it's possible to calculate the velocity. Due to the way the Coriolis effect works at 90° to the movement of an object, the velocities depend on the pressure gradient force acting on the opposite axis.
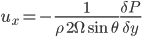
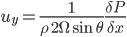
The end result of all of this is that global currents follow the wind (compare Fig. 29 to Fig. 17).
Summary
- Because the earth is a sphere, there is differential heating. The equator receives more solar radiation than the poles. The equation for solar radiation at a latitude is:
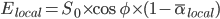
- Differential heating causes winds to develop. Warm air at the equator rises, and cool air from the poles rushes in to replace it. This is the basis for wind.
- The rotation of the earth splits the wind into and three atmospheric cells and three wind belts at latitudes 0°, 30°, and 60°. There are low pressure zones at 0° and 60° where air is approaching and rising. There are high pressure zones at 30° and 90°. Low pressure areas receive more precipitation. The atmospheric cells, in order of increasing latitude and decreasing strength, are the Hadley, Ferrel, and Polar cells.
- The rotation of the earth causes the Coriolis effect, because each latitude spins at a different speed. The equator spins faster than the poles. In the Northern Hemisphere, motion is deflected to the right. In the Southern Hemisphere, motions are deflected to the left. Directly over the equator, the Coriolis effect is 0; it is strongest at the poles.
- The Coriolis effect causes the winds to have east-west components in addition to their north-south components. The wind patterns in the hemispheres mirror each other. From pole to equator, the pattern is EWE (Easterlies, Westerlies, Easterlies). Easterlies travel toward the equator. Winds are named from the direction they are coming from.
- Land-sea differences can change the direction of the winds and cause gyres.
- The pressure on a point in the ocean depends on gravity, height of the water column, and water density. density increases with salinity and decreases with temperature. The water column is stable when less dense fluid overlies more dense fluid; in winter, vertical mixing occurs whereby surface water is cooled, becomes denser, and sinks.
- Due to friction combined with the Coriolis effect, ocean surface currents travel at 45° to the wind. Each successively deep water layer travels at a slightly increased angle to the layer above it. The net transport of water is 90° to the wind; this is called Ekman transport.
- Ekman transport causes causes convergence (or divergence) of water. Convergence causes downwelling; divergence causes upwelling, which brings nutrient-rich deep water to the surface, fueling productivity. Upwelling occurs at the equator at 60°.
- Water in the ocean moves from areas of high pressure to areas of low pressure. Convergence (or divergence) causes high pressure and low pressure zones in deep waters. High pressure and low pressure zones create horizontal pressure gradient forces. Pressure gradient forces cause water to move horizontally from high to low pressure. This horizontal water movement is adjusted by the Coriolis effect until the water travels 90° to the pressure gradient force and 90° to the Coriolis effect. Such a current is called a geostrophic flow.
- The Coriolis effect and pressure gradient force are equal but opposite, because the net acceleration is zero. Otherwise, winds and ocean currents would constantly be gaining speed.
- Due to the balance between Ekman transport, the Coriolis effect, and pressure gradient forces, the ocean currents are the same as the direction of the winds.
- The equations for the balances are:
Hydrostatic Balance: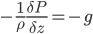
East-West Geostrophic Flow: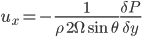
North-South Geostrophic Flow: